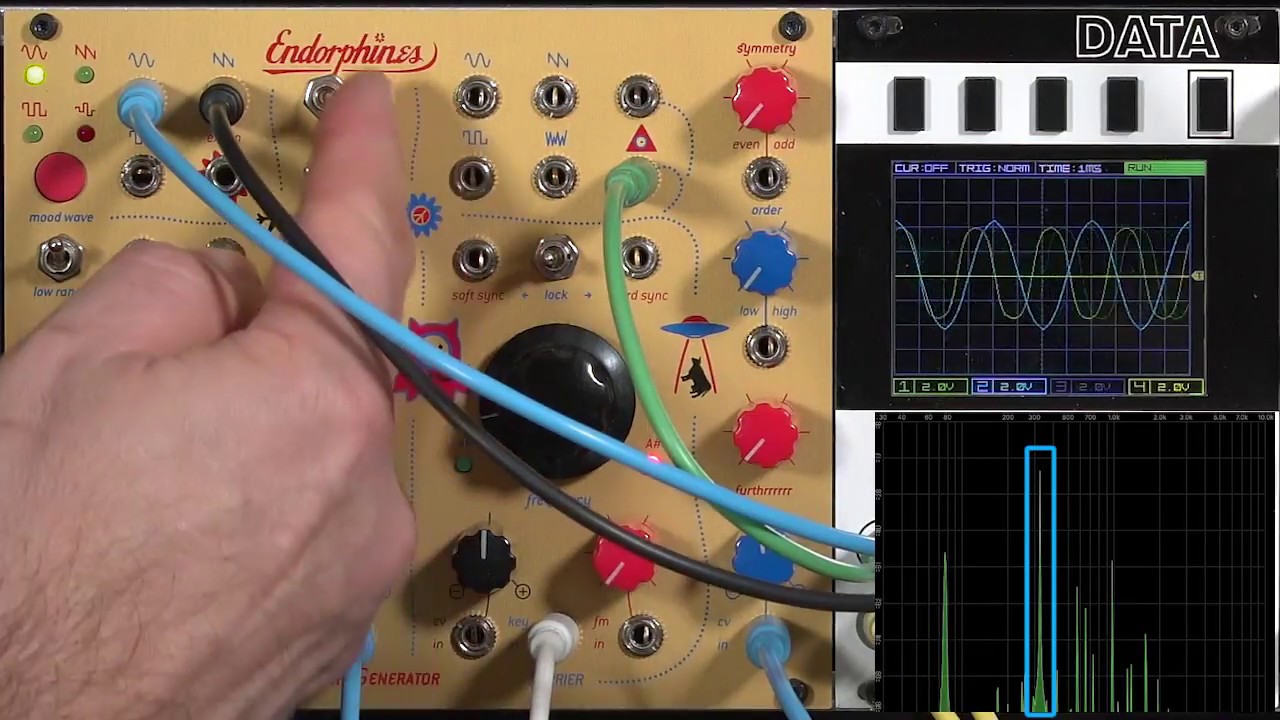
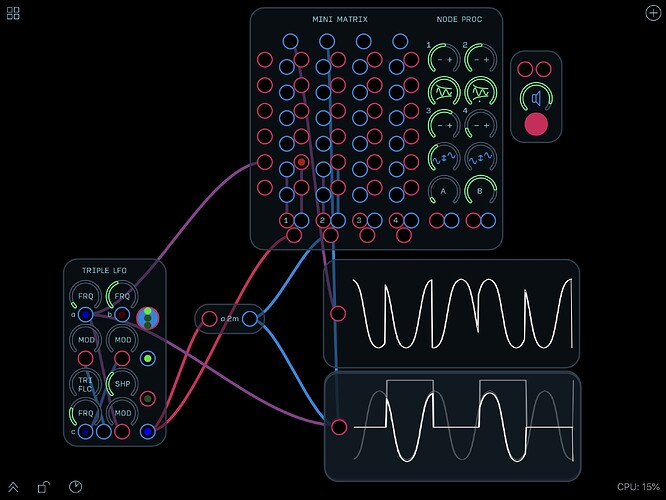
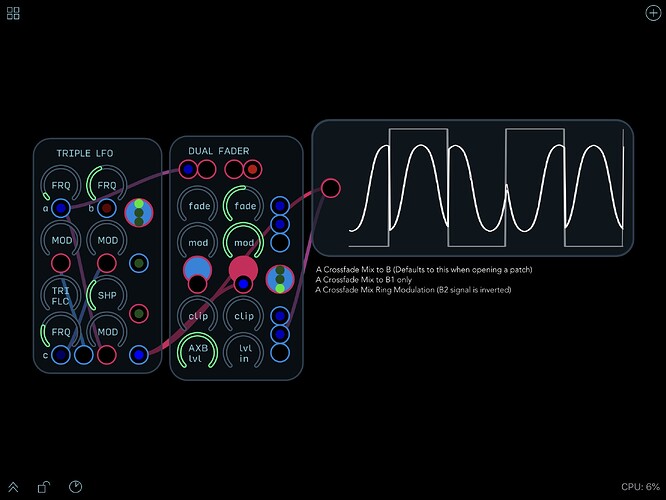
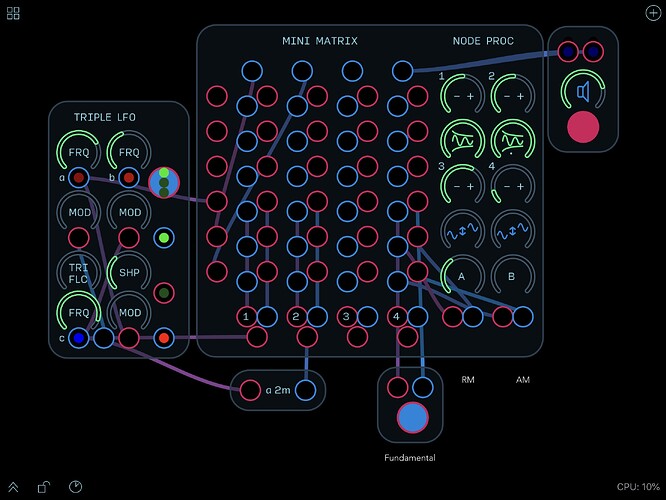
Rob Hordijk includes bipolar VCAs as part of both the Mini Matrix Node Proc and the Dual Fader, and has demonstrated, in the various videos for these modules, the ring modulation effects that this makes possible .
Via a recent Reaktorplayer tweet I came across a nice Learning Modular tutorial demonstrating the difference between amplitude and balanced (ring) modulation.
Towards the end of the tutorial there’s also a nice demonstration of the way in which the fundamental of the carrier falls away with ring modulation but remains present with amplitude modulation.
Following the Learning Modular video, I put together some simple demonstrations using both the Mini Matrix Node Proc and the Dual Fader.
RH-RM Mini Matrix - Node Proc AMBM Demo.audulus (221.9 KB)
RH-RM Dual Fader AMBM Demo.audulus (160.2 KB)
(Use the Dual Fader selection switch to shift between amplitude and ring modulation)
And a version at audio rates: (Knob A to set the level for ring modulation and knob B for amplitude modulation) RH-RM Mini Matrix - Node Proc AMBM (Audio) Demo.audulus (223.6 KB)